Finally, we are can talk about the building block of N-dimension space: The basis. As we learned from the last section, we can linearly combine a set of vectors to form a line, a plane, or even a space. Also, we knew that there is a restriction. Combine these two ideas, we will have our definition of basis:
We called a set of vectors \(\pmb{S}\) is a basis of vector space \(V\) if every element in \(V\) can be written as a unique linear combination of vectors in \(\pmb{S}\) and all the vectors in \(\pmb{S}\) are linear independent.
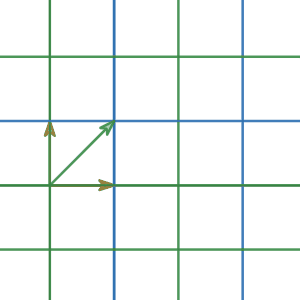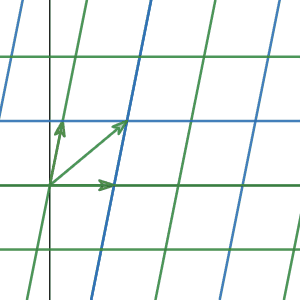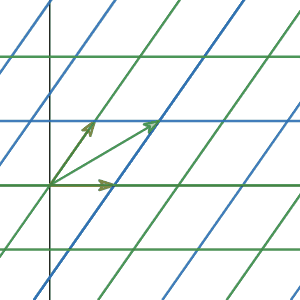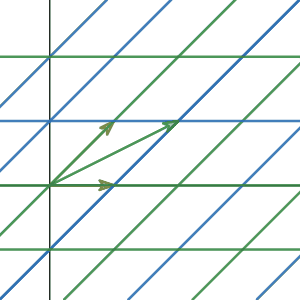
For example, \(set[(0,1),(1,0)]\) is a basis of 2d vector space (real vector space) since any vector in the vector space (a,b) can be written as a unique linear combination of \((0,1)\) and \((1,0)\). Not only that, \((0,1)\) and \((1,0)\) cannot be written as a linear combination of each others, so they are linear independent.
If the set spans the vector space but the vectors in it are not linear independent. We can still call them the generating set or the spanning set of vector space. The name comes from the fact that the vectors “generate” the whole space.
In linear algebra, we can use the basis to represent the corresponding vector space. But what is the actual difference between two different basis if they describe the exact same vector space?
We can construct the 2d Cartesian plane use \(set[(0,1),(1,0)]\), we can also do the same thing with \(set[(1,1),(1,0)]\). So what is the point of writing vector space in terms of different basis? Is it just for fun?
The answer is of course not. In fact, this idea of presenting vector space on a different basis is a huge topic in linear algebra. Change the basis of a vector space will most of the time simultaneously change all the vectors in that space. To understand how the vector change in space, we have to learn the “essence” of linear algebra: the linear transformation.